2.37

练习 2.37 假定我们将向量 v=(v_i) 表示为数的序列，将矩阵m=(m_ij)表示为向量（矩阵行）的序列。例如，矩阵：

$$\left[ {\begin{array}{cc} 1 & 2 & 3 & 4 \\ 4 & 5 & 6 & 6 \\ 6 & 7 & 8 & 9 \end{array} } \right]$$

用序列((1 2 3 4) (4 5 6 6) (6 7 8 9))表示。对于这种表示，我们可以用序列操作简洁地表达基本的矩阵与向量运算。这些运算（任何有关矩阵代数的书里都有描述）如下：

(dot-product v w) 返回和 $\sum_i v_i w_i$ ；

(matrix-*-vector m v) 返回向量 t，其中 $t_{i} = \sum_j m_{ij} v_j$ ；

(matrix-*-matrix m n) 返回矩阵 p，其中 $p_{ij} = \sum_k m_{ik} n_{kj}$ ；

(transpose m) 返回矩阵 n，其中 $n_{ij}=m_{ji}$ 。

我们可以将点积 (dot product) 定义为：

(define (dot-product v w)
    (accumulate + 0 (map * v w)))

请填充下面过程里缺失的表达式，它们计算出其他的矩阵运算结果（过程 accumulate-n 在练习 2.36 中定义）。

(define (matrix-*-vector m v)
    (map <??> m))

(define (transpose mat)
    (accumulate-n <??> <??> mat))

(define (matrix-*-matrix m n)
    (let ((cols (transpose n)))
        (map <??> m)))

首先，定义 accumulate

(define (accumulate op initial sequence)
    (if (null? sequence)
        initial
        (op (car sequence)
            (accumulate op initial (cdr sequence))) 
    )
)

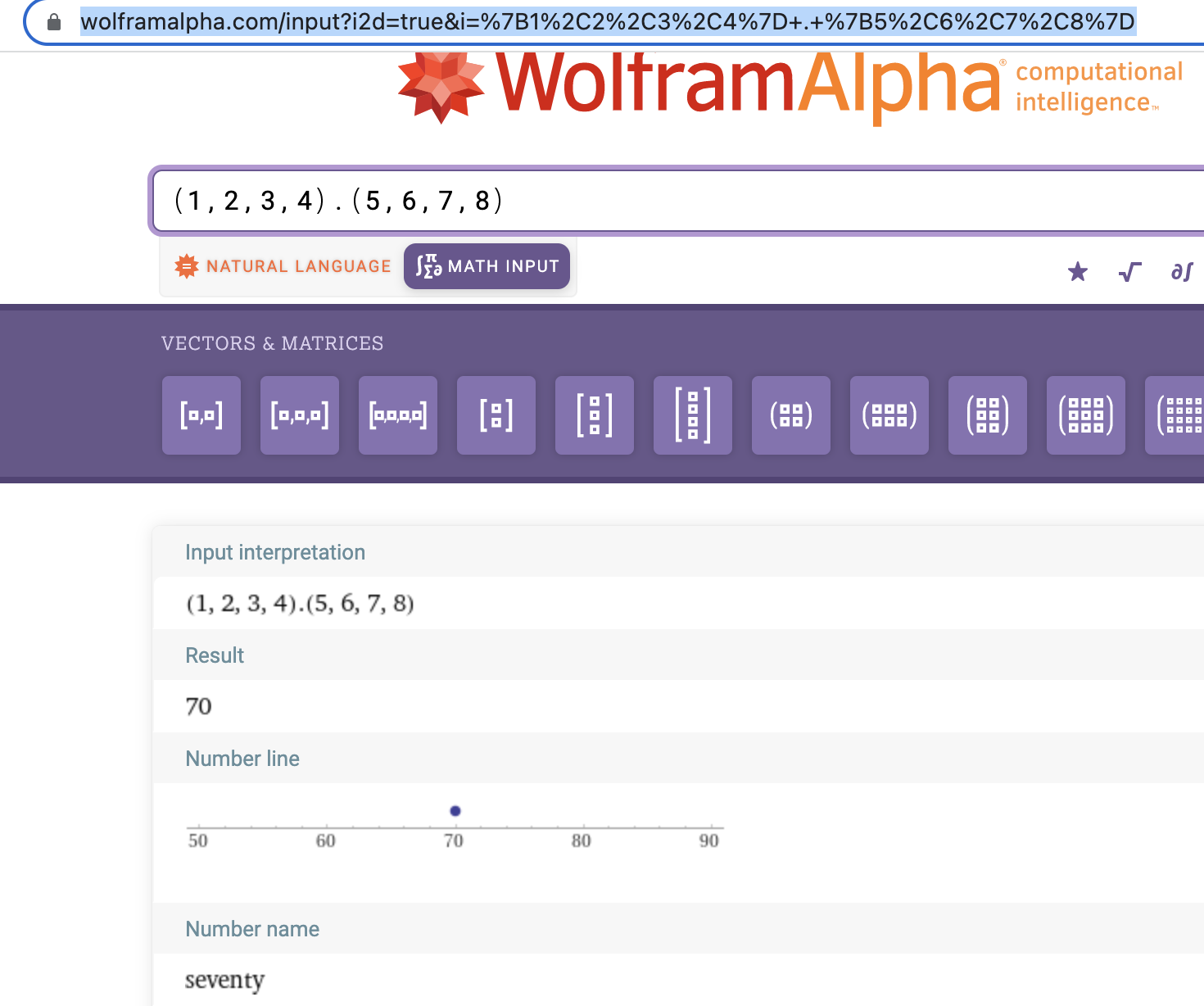
(define (dot-product v w)
    (accumulate + 0 (map * v w)))

(dot-product (list 1 2 3 4) (list 5 6 7 8))

对比:

(define (matrix-*-vector m v)
    (map (lambda (m_i) (dot-product m_i v)) m))

(define m (list (list 1 2 3 4) (list 4 5 6 6) (list 6 7 8 9)))
(define v (list 1 2 3 4)) 

(matrix-*-vector m v)

对比：

然后，再定义 accumulate-n

(define (accumulate-n op init seqs)
    (if (null? (car seqs))
        '()
        (cons (accumulate op init (map car seqs))
                (accumulate-n op init (map cdr seqs)))))

(define s (list (list 1 2 3) (list 4 5 6) (list 7 8 9) (list 10 11 12)))
(accumulate-n + 0 s)

(define (transpose mat)
    (accumulate-n cons '() mat))

(transpose m)

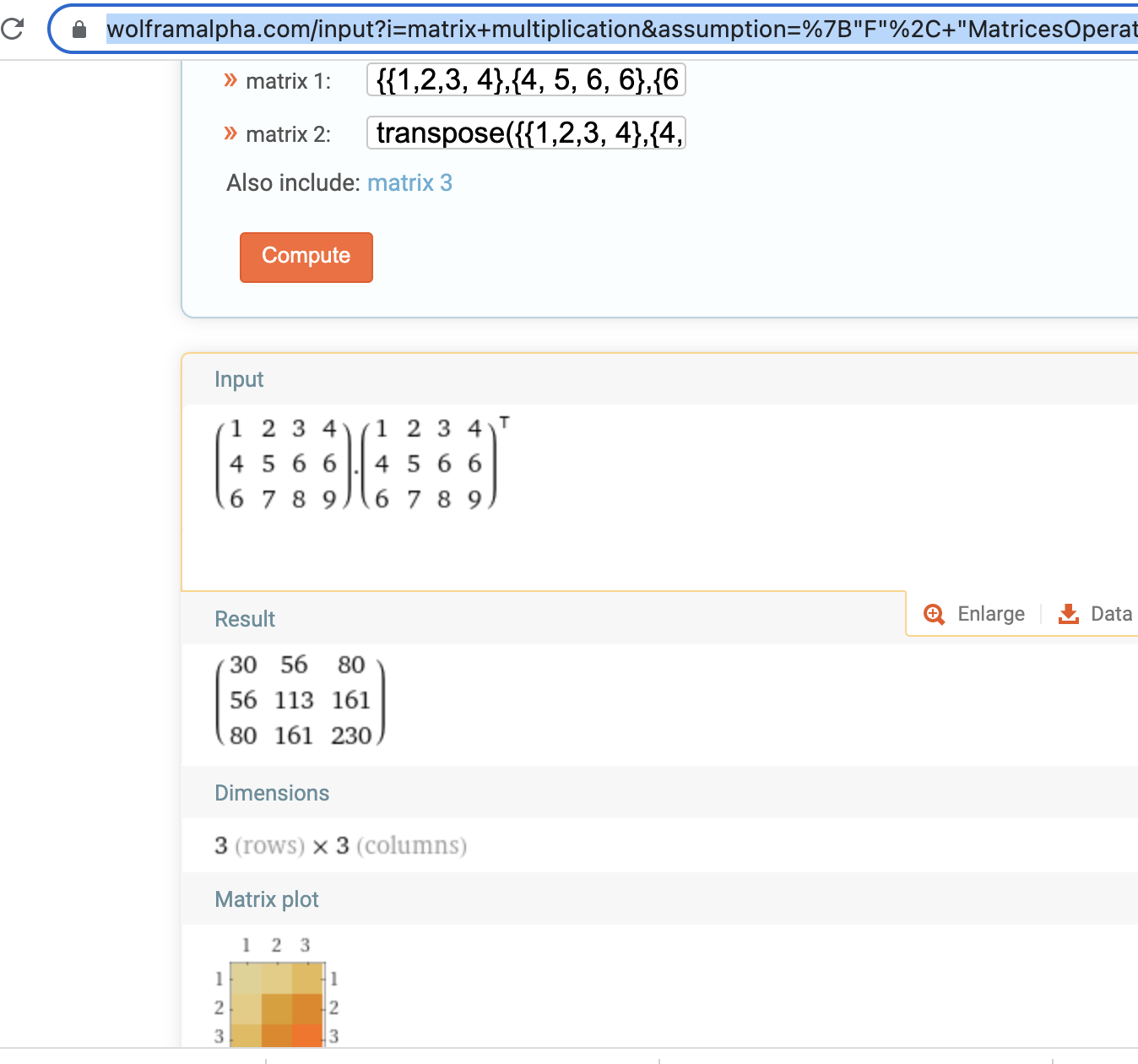
(define (matrix-*-matrix m n)
    (let ((cols (transpose n)))
        (map (lambda (v) (matrix-*-vector cols v)) m))) 

(define n (transpose m))

(matrix-*-matrix m n)

对比：